When two or more images are spliced together , to create high quality and consistent image forgeries, almost always geometric transformations such as scaling, rotation or skewing are needed.
The knowledge of whether the given image or some of its portions have been resampled can play an essential role in many fields, especially in image forensics. When two or more images are spliced together, to create high quality and consistent image forgeries, almost always geometric transformations such as scaling, rotation or skewing are needed. Geometric transformations typically require a resampling and interpolation step. Therefore, by having sophisticated resampling/interpolation detectors, altered images containing resampled portions can be easily identified and their successful usage significantly reduced.
Despite the importance, massive usage and history of interpolation, to our knowledge, there exist only a few published works concerned with the specific and detectable statistical changes brought into the signal by this process. In this work we analytically described specific periodic properties present in the covariance structure of interpolated signals and their nth derivatives. Without the detailed knowledge of how the statistics of the signal is changed by the interpolation process, applications based on statistical approaches working with resampled/interpolated signals or with their derivatives can yield miscalculations and unexpected results.
Furthermore, we proposed a blind, efficient and automatic method capable of detecting the traces of resampling and interpolation. The proposed method is based on a few main steps:
-
ROI selection,
-
signal derivative computation,
-
radon transformation
-
search for periodicity.
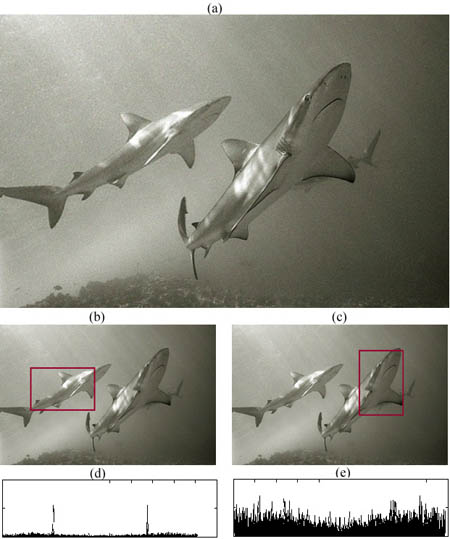
The above example shows an example of the method's output. Shown are: an image containing a resampled region (a). In this image, the shark on the left side has been resized by factor 1.4 using the bicubic interpolation. Output of the method is shown in (d). Peaks clearly signify the presence of interpolation. The method has been applied to the denoted region shown in (b). The output of the method applied to a non–resampled region is shown in (e). The tested region is shown in (c). Obtained results show that it is possible in a simple and fast way to find traces of general affine transformation when a low order interpolation polynomial has been used.
Contact person: Babak Mahdian



